Probabilistic material and component assessment

Jump to:

Probabilistic Material and Component Assessment

Methods and Activities

Publications

In engineering practice, the design of a component is normally ascertained based on deterministic material data and component loading scenarios. The considered loads are based on experiences, measured data and load scenarios covering a whole variety of possible service load cases. Minimum values from standards or measurements are generally used as material properties. Such a procedure delivers information concerning whether a component can be operated in service, also incorporating an additional safety margin. However, it is not taken into account that, in reality, deviations from the assumed input data may occur with a certain probability. Such deviations may be caused by e.g. scatter of material properties, disordered microstructures, defects in materials, deviations from the given construction, manufacturing defects, unknown internal stresses such as residual welding stresses or unexpected external loads. The aim of a probabilistic component assessment is to account for the probable occurrence of such deviations in the component integrity assessment as well as to predict the component behavior and its reliability. Often, such reliability checks are essential both for the risk analysis and to avoid violations of warranty statements.

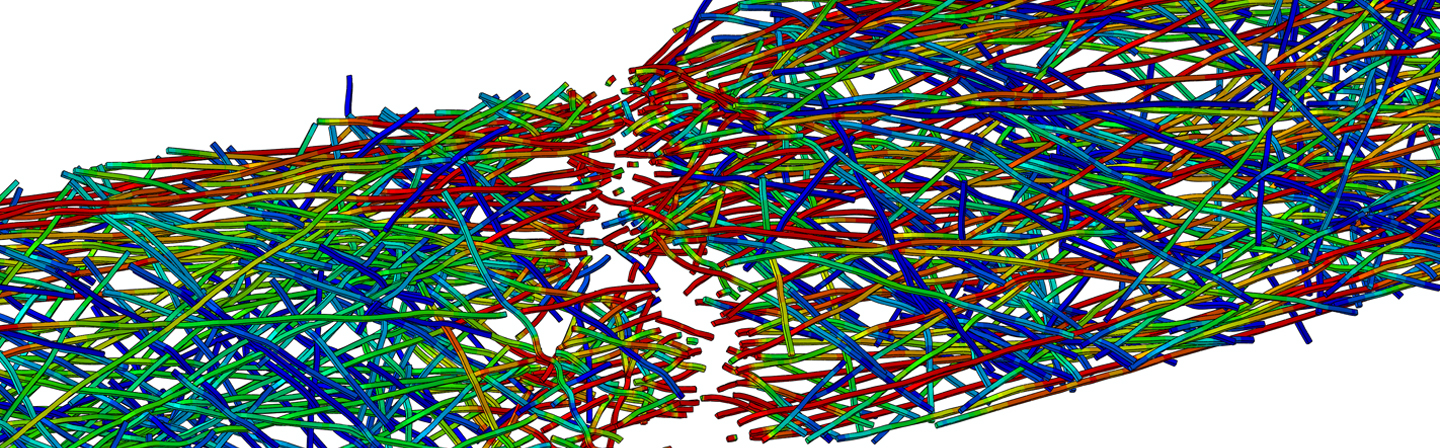
The research and development work at Fraunhofer IWM focuses on the probabilistic material and component assessment of modern materials such as fiber-reinforced materials, polymeric or metallic foams, high-strength steels, casted irons or steel alloys as well as weldments. In these materials, which are applied in various industrial sectors – from the automotive and aviation sector to medical components – differences in their microstructure or existing micro or macro defects have a significant influence on strength features. By means of probabilistic analyses, heterogeneous microstructures and the related property scatter can be considered to achieve a comprehensive safety and reliability assessment.

to top 

• Experimental microstructure characterization to determine distinguishing material parameters and their probability of occurrence (e.g. cell or grain sizes, fiber lengths, orientations, defects)
• Randomly controlled microstructure generation based on statistically representative input values considering their scatter:
  • Cell structures (open or closed cells)
  • Fiber reinforcements
  • Grain structures, distributions
• Probabilistic homogenization to derive macroscopically effective material properties (e.g. stiffness), their scatter as well as their spatial correlation by means of statistically representative volume elements and cross-checks to verify that these volume elements are collectively representative for the material
• Effective calculation by means of specific discretization of the random space instead of pure Monte-Carlo simulation
• Derivation of probabilistic constitutive equations and algorithm development for the generation of random fields for probabilistic component calculations considering microstructure fractions, their scatter and correlation
• Validation by means of experimental and numerical, static/cyclic, micro and macro tests
  • Determination of effective material properties and stress-strain curves
  • Local strain field measurements, digital image correlation (DIC)
  • Evaluation of size effects
• Evaluation of crack initiation and growth by means of coupled damage and fracture mechanics methods for components with defects
• IWM VERB - a software tool for the assessment of components with crack-like defects
• Probabilistic methods to determine the distribution of load cycles to crack initiation

to top   

• Hohe, J., Geometry modelling and elastic property prediction for short fiber composites, Multi-scale continuum mechanics modelling of fibre-reinforced polymer composites, Part I: Geometry construction and homogenization of linear elastic material behavior at micro- and meso-scale; van Paepegem, W. (Ed.); Elsevier B.V., Amsterdam, NL (2021) 55-77 Link
  
• Radaelli, F.; Amann, C.; Aydin, A.; Varfolomeev, I.; Gumbsch, P.; Kadau, K., A probabilistic model for forging flaw crack nucleation processes, Proc. of ASME Turbo Expo: Power for Land, Sea, and Air 2020, Vol. 10B/Structures an Dynamics; American Society of Mechanical Engineers (ASME), New York, NY, USA (2021) Paper No: GT2020-14606, V10BT28A004; 10 pages Link
• Hohe, J.; Beckmann, C.; Böhme, W.; Weise, J.; Reinfried; M.; Luthardt, F.; Rapp, F.; Diemert, J., An experimental and numerical survey into the potential of hybrid foams, Mechanics of Materials 136 (2019) 103063 1-15 Link
• Zerbst, U.; Madia, M.; Schork, B.; Hensel, J.; Kucharczyk, P.; Ngoula, D.; Tchuindjang, D.; Bernhard, J.; Beckmann, C., Fatigue and fracture of weldments - The IBESS approach for the determination of the fatigue life and strength of weldments by fracture mechanics analysis; Springer Nature Switzerland AG, Cham, Schweiz (2019) 157 Seiten Link
• Beckmann, C.; Kennerknecht, T.; Preußner, J.; Farajian, M.; Luke, M.; Hohe, J., Micromechanical investigation and numerical simulation of fatigue crack formation in welded joints, Engineering Fracture Mechanics 198 (2018) 142-157 Link
• Hohe, J.; Paul, H.; Beckmann, C., A probabilistic elasticity model for long fiber reinforced thermoplastics with uncertain microstructure, Mechanics of Materials 122 (2018) 118-132 Link

to top